The Value of Thinking for Satisfying our Quest for Knowledge
GA 164
20 August 1915, Dornach
Translated by Steiner Online Library
Episodic Observation On Space, Time, Movement
I thought that at most a dozen people would be here today and wanted to say something, as is to be expected, that is not part of our usual considerations, but which may be important for some who can immerse themselves in the matter, for the assessment of some things that currently play a role in relation to certain concepts of space and time and movement.
There are theoretical physicists today who believe that a profound revolution is taking place in relation to the simplest conceptions of the world. Among these simple conceptions of the world, on which theoretical physics is based, we want to look today at a small part that relates to time, space and motion. This will provide the basis for a more extensive consideration to be undertaken in the near future, which can lead us deeper into what is currently being sought in fundamental physical considerations.
You will all have heard that what is known as the theory of relativity is prevailing in modern physics. The theory of relativity – and here there are also many variations – is today advocated by countless theoretical physicists. It is expected to bring about a complete revolution in all the concepts that physicists, when they have been engaged in elementary theoretical considerations, have hitherto recognized as correct and which, after all, go back essentially to Newton. Now, however, the newer theoretical physicists of today believe that all these Newtonian concepts, which were still accepted as absolutely incontrovertible during our time as students, must undergo a revolution, indeed that, to a certain extent, the entire theoretical basis of physics, as it has been believed and is still believed, is actually false. Now, the reason why I have to relate the observation I want to make to this newly emerging theory of relativity will become clear later.
In order for what I have to say not to remain completely incomprehensible, I would like to start from very simple, elementary concepts in order to show you immediately what kind of idea can be associated with the concept of time. Let us start, as I said, with very elementary things. Let us assume that some object, which I will call \(a\) for the sake of argument, a rolling ball or something similar, moves in a direction that I will indicate with this line; so \(a\) moves along the straight line in the direction of \(b\):

Now, as you all know, the distance traveled by such a moving object in one second is called the speed. So let us assume that \(a\) would come here in one second, up to \(a_1\). In physics, this distance \(a\) up to \(a_1\) would be called the speed and denoted by \(c\). And if we further assume that the moving object continues through the following seconds, then, if it were to perform a uniform motion – and we only want to talk about such a motion – it would be at \(a_2\) at the end of the second second, where \(aa_1 = a_1a_2\) , that is, with the same speed \(c\). During the second second, the moving object goes from \(a_1\) to \(a_2\), during the third second from \(a_2\) to \(a_3\), during the fourth second from \(a_3\) to \(a_4\), and so on. Now, let us assume that we observe this movement for a certain period of time and that our movable object travels a certain distance. If it moves from \(a_5\)
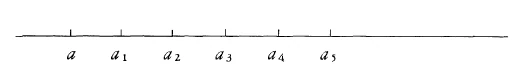
then, when this movable has rolled from \(a\) to \(a_5\), the piece of space - which we understand here in its one dimension - is called the way; so that \(a\) to \(a_5\) is the way that it has traveled; \(c\) is the speed; the distance traveled is denoted by \(s\); and one says: the movable \(a\) has traveled the distance \(s\) at a speed \(c\) in a certain time - here five seconds. This transit time is denoted by \(t\).
Now there is a certain relationship between distance, time and speed. The simplest relationship that has been found is that one would say here: \(s\) - the distance - is five times from \( \)to \(a_1\), that is, once the distance \(a\) to \(a_1\) times \(5\), which is \(5\) seconds, so that is the time ; so we have to multiply what we have called the speed – this piece of \(aa_1\) – by \(5\), and then we get the distance \(s = c \cdot t\) (distance = speed \(\cdot\) time). So there are three terms in this formula: \(s\), \(c\), \(t\).
Now you know that an infinite amount has been written about time by a number of philosophers, mathematicians and also theoretical mechanics. People believe that they have an idea, a concept, of time, but if they had to explain and reflect on what they understand by time, everyone would very soon realize that they do not really have a proper idea of this concept of time, which is one of the most commonly used concepts in mechanics. In order to be able to study anything about the concept of time, let us stick to this formula, which, after all, initially sets the concept of time into a uniform, rectilinear motion. But even though this formula can be found in every physics book, in physics it is surrounded by a whole host of – I won't say ambiguities, but a lack of clarity, a lack of will to go deeper into the matter. And this is due in particular to the fact that in our schools, the teaching with regard to something that we all learn does not teach us certain distinctions, but these are important if one wants to arrive at more precise concepts in a certain direction. In our schools, we learn to speak of four types of calculation: addition, subtraction, multiplication and division. But when it comes to division, I don't think we are often made aware that there are actually two completely different things involved in the usual calculation operation. I will show you this in a very simple way.
Let us assume that we have an ordinary apple and divide it. We can divide it into five, into ten parts and so on, then, when we have divided it, we get a so-and-so-many-parts of the apple. If we want to distribute the parts, what we distribute is just a piece of the apple. We are actually performing a division here. I want to write it as a fraction, because that is the same as a division. I can say: an apple is divided into, say, ten parts, and the result is one tenth of an apple. Now take a look at what I have written on the blackboard:
$$\frac{1 apple (thing)}{10 (number)} = \frac{1}{10}apple (thing)$$In the numerator or dividend, we have a quality, something real; in the divisor or denominator, we have nothing real, but a mere number; \(10\) is a mere number here; and in the quotient, we have something real again: one tenth of an apple.
This fact does not change if we divide twenty apples instead of one. If we divide \(20\) apples by \(10\), we get \(2\) apples instead of one tenth of an apple:
$$\frac{20 Apfel}{10} = 2 Apfel$$The \(20\) apples are again a thing; below is only the number and as a quotient we get again a thing. That is a division.
But dividing can have a completely different meaning. I can have \(20\) apples in the dividend above, but below as the denominator or divisor, let's say \(2\) apples, then I have a thing above and below. What do I get as a result? In this case, I do not get a real thing as a result, but I find out how often \(2\) apples are contained in \(20\) apples, I get \(10\), that is, I get a number:
$$\frac{20 apple (real thing)}{2 apple (real thing)} = 10 (number)$$Again, I am dealing with a division, but this time it has a completely different meaning than the division in the first case. In the first case, I divide a thing and get another thing in return; in the second case, I don't divide at all, but set myself the task of exploring how often a thing is contained in another thing, and that's where I get a number.
We can therefore say that division is not always the same as dividing, but that there are two types of division that are strictly different from each other. When teaching, it is therefore always necessary to explain that there are two types of division. In the first, the task arises for me to investigate what comes out when you divide a thing; in the second, the task arises to investigate how often a thing is contained in a similar thing – they must be similar, because of course you can't ask how often \(2\) apples are contained in \(20\) pears – and then we get a number out.
This must be borne in mind when studying the formula \(s = c \cdot t\).
Now this formula can also be written differently. I don't always have to look for the \(s\), but I can also look for the \(c\) or \(t\), then the formula changes. If I look for the \(c\), then I get it by dividing the \(s\) by \(t\). By dividing the whole space by \(t\), I get the space that has been traversed in \(5\) seconds, by \(5\), which is the speed \(c\): $$c= \frac{s}{t}$$ Likewise, you can get \(t\): the time. Let's assume that you divide \(s\) by \(c\). If you ask: how often is the distance of one second included in the whole distance, then it is included five times. So you get the time:
$$t = \frac{s}{c}$$Let's take a closer look at these formulas. First, let's take the second one and compare: \(s\), which is the distance here, the length \(a\) to \(a_5\), we have that in the numerator; here in the denominator we have \(c\). What is \(c\)? Well, that's the distance in one second. Distances are this: \(s\) is a distance, \(c\) is a distance. What form of division does that resemble? Well, it resembles this form \(20\) apples : \(2\) apples = \(10\). Here (in the numerator) you have apples and here (in the denominator) you have apples; here (in the numerator of \(\frac{s}{c}\)) you have distance and here (in the denominator) you have distance. What should be in front of that? Just a number. That is, \(t\) turns out to be nothing more than a number in our physical considerations. For if I regard \(s\) and \(c\) as distance, that is, as a thing - both are, after all, distance or a piece of distance -, then, from the nature of the division, time \(t\) can only figure as a number. Just as the number \(10\) (\(20\) apples : \(2\) apples = \(10\)) is a number and nothing less or more, so in this division \(t\), time, can also be nothing but a number.
You can also take the division form \(1 apple: 10 = \frac{1}{10} apple\), then this is the same as the formula \(c = \frac{s}{t}\). On the other hand, if we divide a thing by a thing, what must come out? A number like here \(t = \frac{s}{c}\), where with \(t\) we are dealing with a mere number. That is, both formulas indicate that - insofar as we stop at physics - we get nothing more than a number for the time after the nature of the division. And in this case (\(20\) apples: \(2\) apples = \(10\)) it is a number that refers to apples and shows how often \(2\) apples are contained in \(20\) apples, and here, in the case of time, \(\frac{s}{c} = t\) is a number that shows how often the velocity is contained in space.
Now, none of you will see the number as such as a thing. If you give any boy or girl not \(3\) apples, but only \(3\) as a number, they will not be satisfied. So in the number you cannot see a thing, but just a mere abstraction, something that merely indicates relationships in the external world.
From this consideration, we can see that time itself slips through our fingers through the physical consideration; it shrinks to a mere number. Just as we cannot philosophize about the number, we cannot philosophize about time either, that is, it has been reduced to the idea of a number. That is why we cannot find time in things, no matter how long we search everywhere, because it only appears as a number. What is the connection? Well, I don't think a boy or girl needs to be particularly old to give a healthy gut feeling answer to the question: What interests you, the apples or the number? Of course, someone could speak sophistically and say, “I am interested in the number because I prefer \(8\) apples to \(6\)”; but that is only because \(8\) apples are more than \(6\). So the number is not what it is about, but the apples are, the tangible is.
But from this it follows that we must adhere to the material and not to the number when we speak of time, space and speed. And if we now consider the material, time is eliminated from the outset, that is, it is a number and not a material thing. So you can say to yourself: we have \(s\), we have space, we have the piece of space that our movable passes through. If it continues to roll, it can still cover a great deal of space. Space is something material outside. But that is not what is most important, because space can be thought of as continuing forever. But there is something else that is very important to us, and that is \(c\). How \(a\) travels through space depends entirely on whether it travels, say, \(20\) or \(25\) or \(50\) cm in one second, and so on. In turn, how much it travels depends on how fast it moves. But how fast it runs, that is inside it, that is peculiar to it inside. And the whole process depends on what is peculiar to the movable inside. So it depends on the speed of the movable, which belongs to the movable as such, is an inner quality of the movable. And if we look at the world in terms of mechanical processes, then, when we speak of reality, we must speak of the intrinsic speed of the bodies or atoms or molecules. And the whole process forces us to speak of intrinsic speed as belonging to things, just as red color belongs to the rose.
So the fundamental concept is speed; it is what matters. It follows that we must not adhere to the formula that has \(c\) here \(c = \frac{s}{t}\), and must not believe that with space and time we have something particularly real, but what is real in things is speed, not time. Time, in turn, is only abstracted from the concept of speed because things have different speeds. If we look at the different speeds and want to reduce them to a common denominator, we get the concept of time. This is an abstraction, just as the generic term “apple” is an abstraction and only the particular, the concrete apple, is real. So if we look at the mechanical reality of things, we have to look at speed and must not believe that we can put the concept of time in the foreground. That is the big mistake that is made everywhere in physics, that one does not consider that one must start from the speed that is inside things, that belongs to them as life belongs to living bodies.
So, my dear friends, remember: not time but velocity is what must underlie mechanics. You might say that making these distinctions is mere madness. But it is not madness. These things are fundamental to our understanding of certain aspects of reality, and I will point out to you in a moment something that shows how fundamentally significant they are.
In the various discussions about the theory of relativity, people were struggling to come to terms with the concepts of time and speed. Now I would like to show you, by means of two speculations, the way in which certain people think and formulate their thoughts when they talk about time and speed. I have to introduce you to a strange character, Mr. Lumen, who plays a certain role in the theory of relativity. What kind of strange gentleman is he? Yes, you see, this is an, I would say, “imaginary acquaintance” that Flammarion has made. This Mr. Lumen has a very strange ability, which we can understand in the following way.
You all know from your physics lessons that light has a certain speed; it travels 300,000 km per second. \(c\), so everything that, in our view, is mechanically inherent in light is a speed of 300,000 km per second. Let us assume, for example, that here is the earth and that a beam of light goes out into space from the objects and events that happen on earth (as schematically indicated on the board) and one says yes, because the light goes out, one sees the things. Let us now assume the following. We have had a somewhat abstruse mathematical-physical lesson, and, let us say, a eurythmy lesson from three to four o'clock. From all this, the light goes out into space and one can observe from outside what is happening here. And since light travels at a speed of 300,000 km per second, what happened here between three and four o'clock this afternoon also went out into space at a speed of 300,000 km a second, so that if you imagine an observer 300,000 km away, he will see what is happening here on Earth only after one second.
Now Flammarion assumes that Mr. Lumen rushes out into space even faster than the speed of light, namely at a speed of 400,000 km per second. What will be the result of this? He will continually overtake the light, because after the light has traveled one second, he is already 100,000 km further away. When he rushes out and looks back, he must come to the manifestations of light, where he sees what has happened here now and between three and four o'clock. But since he not only catches up with the light, but overtakes it, it must follow that he does not perceive the eurythmy lesson first and then our lesson, but everything in reverse, first the end and then the earlier. It is a strange spectacle that this Mr. Lumen experiences. He sees everything in such a way that he first sees the end and then the beginning, because he overtakes the light.
As I said, such ideas have played a certain role in the discussions about the theory of relativity. I would like to present you with yet another idea, which has also played a certain role and which the naturalist Baer formed. He said to himself: One could imagine that man does not live his life in about 70 or 80 years, but in 70 or 80 seconds. His pulse would simply have to beat so much faster that one second would contain a year. This could cause man to be not just like a mayfly, but like a 70-second animal, if only his pulse beat fast enough. What would be the result? Such a person would experience tremendous things in 70 seconds. If, for example, he looks at a plant that has remained true to its species, he would never come to the conclusion that a plant grows out of the earth, but he would come to the conclusion that plants are eternal beings. Thus, such a person would have a completely different view of the world, simply because the speed of his life could be thought of as increasing in the same measure as the speed of his pulse compared to the rest of us. Or, says Baer, let us imagine that man does not live 80 seconds or 80 years, but 80,000 years, and that the pulse rate is so much slower, then the whole world would be different again. For example, the sun, which appears to move at a certain speed to us, would race across the sky like a fiery wind; we would not distinguish the individual sun, but would see it racing around like a reddish wheel. Plants would shoot up quickly and then fade away at breakneck speed, and so on.
Baer presents this as a possible idea to show how the world view depends on the subjective constitution of the organism. You see, everything, absolutely everything, is called into question.
If we consider the type of thinking that underlies such ideas as those of Mr. Lumen or Baer's Flammarions, one thing is important to note. Let's take Mr. Lumen again. It is assumed that Mr. Lumen would be able to fly 400,000 km per second, thus overtaking light and catching up with later light images. But now take what you can really take when you delve deeper into our spiritual scientific concepts. We can even disregard the coarser physical body altogether and go straight to the etheric body. Yes, when we go into the etheric body, what is it? It is ether, light ether, it is itself weaving light. Hold on to that, because what follows from it? It follows that when we move in space, we can move at the highest speed that is peculiar to light. So if someone says that a person like Mr. Lumen moves at a speed of 400,000 km per second, then we have to ask – I will even leave out the physical body and just assume that an etheric body could move out – how fast could he possibly move? Well, at most at a speed of 300,000 km per second, the speed of light. We cannot say that the etheric body overtakes light, because it is itself movable light. So Mr. Lumen cannot be woven out of anything that exists in space; in other words, he is an unreal conception, he is a pure fantasy. For to the tangible or the substantial in the world, its speed is immanent or inherent. It is inside of it. It is its property. We cannot tear it out. We cannot say: we separate the speed from the thing – but it is a property of the thing. We cannot speak of a property that exists separately outside the thing. So we must also say, in the face of Baer's ideas: in the moment when one realizes that the speed of the pulse beat belongs to the essence of every human being, one also realizes that we cannot have any other speed than that of our pulse beat. We are human because we have a certain pulse rate, and we cannot imagine it being any different, because we would cease to be human if, for example, our pulse were a thousand times faster than it actually is. Speed belongs to the material world.
It is important to see how spiritual science leads to the essence of things, and what the thinking that has developed into our time leads to without engaging in spiritual science. It leads to the formation of ideas such as those of Mr. Lumen or the pulse rate that has accelerated a thousand times, which are simply impossible or unreal. One calculates with fantastic concepts if one does not realize that time is a mere number. Thus, so-called rational mechanics has led to completely unreal concepts. Spiritual science leads us to say: Yes, what is such a Mr. Lumen, who races 400,000 km while he would at most travel 300,000... [gap in the transcript]... He is nothing more than the famous gentleman who pulls himself up by his own hair.
From this point of view, then, spiritual science is there to bring human thinking, which has lapsed into fantasy, back to reality, not to dissuade it from reality. You see, while people accuse spiritual science of being fantastic, it is actually there to guide the fantastic ideas and concepts of physics back to reality. And it will be extremely important for healthy thinking in the future that children are really taught something like the two types of division, so that they do not calculate with all kinds of ambiguities, but with definite concepts. When it comes to ideas and concepts that have a bearing on reality, there is no other way than to face reality squarely, that is, to think with spiritual science, because only then do real, not fanciful, concepts emerge.
Before the theory of relativity, physics had Newton's idea that space is an emptiness, a vessel, so to speak – whether it is infinite or not, we will not examine that now – and time flows like a uniform stream; things are in space and processes run in time, and depending on whether a thing needs this or that time to cover a certain space, it is given a certain speed. This idea is untrue because it does not look at the essence of space and time and thus divides speed, which is actually an inner property, into the two unreal ideas of space and time. Speed is truly the original, while physics always regards speed as a function of space and time. But what belongs to things is their essential nature, and spiritual science shows that one must take certain paths in order to avoid fantasies about space and time - such as that of infinite space or that of time as a flowing stream - but to arrive at the real reality of speed. The entire field of mechanics, which we absorbed in our youth as something tremendously secure, as the most secure there is in science after mathematics, operates with very vague concepts because it does not know what the nature of speed is and does not know how to regard it as fundamental.
Now the impetus for the theory of relativity from Minkowski, Einstein, Planck, Poincaré, the late mathematician and physicist and so on, came precisely because they could no longer cope with this childish Newtonian idea of empty space and regularly flowing time and things that move at a certain speed. Certain experiments led to concepts that did not agree with what had been considered the most certain.
Recently, I have developed a concept here that is purely related to spiritual science, which may have come as a surprise to some. I have developed the concept that it is not true at all to believe that the most important thing in the head is substance, matter, because precisely where we suspect matter, it is hollow and, from a spiritual point of view, we are all hollow-headed. I used the comparison with the air bubbles in a bottle of Selters water. There it is also the case that where we believe we perceive something real, there is nothing. All around is the spiritual reality and in it there are holes everywhere; you see them, just as you only see the bubbles in the Selters water, which are air, you do not see the water. And if people believe that there is something where I touch the table, that is not true either, because there is actually nothing there. I touch the hollow space and because there is nothing there, that is why I cannot go further.
We arrived at this conclusion quite systematically on the basis of spiritual scientific premises. Certain insightful and perceptive physicists have now been pushed to a similar conclusion by other means, because certain processes in nature simply do not agree with the concepts of Newtonian mechanics, which are considered so certain. And these things include, for example, the processes at work in the cathode rays that you are familiar with, which, as you know, can be observed in certain evacuated glass tubes. Here we are dealing with something that, as a moving part, has speed, with electrons, figuratively speaking, with flowing electricity. And through observation, through the experiment of observing the cathode rays in the tubes, which are flowing electricity, the physicists have come to very peculiar ideas. And I would like to read you one such idea. It can be found in a lecture by Poincaré on “The New Mechanics”. He ties in with the ideas that arise from the cathode ray experiment, because this does not agree with the Newtonian concept of speed. And after rather confused trains of thought, he sees himself compelled to make the following concession:... [gap in the transcript] ..., and there the physicist feels compelled to say the following:
“Matter has now become completely passive. The property of resisting the forces that seek to change its motion no longer applies to it in the true sense of the word. When a cannonball moves at high speed and thereby becomes the carrier of a living force, of a tremendous energy that spreads death and destruction, it is no longer the iron molecules that are the seat of this energy, but this seat is to be sought in the ether that surrounds the molecules. One could almost say that there is no longer any matter, only holes in the ether.” - Well, what more do you want, my dear friends? — “And as far as these holes seem to play an active role, it consists in the fact that these holes cannot change their location without affecting the surrounding ether, which reacts against such changes.”
Matter is holes in the ether! Physics is therefore compelled to admit this based on its current experience. And building on such experiences, another physicist, Planck, uttered a sentence that is highly remarkable, namely the sentence that says: We experienced in the forties of the 19th century that Helmholtz approached a certain problem in such a way – it wasn't Helmholtz, but Julius Robert Mayer, but we don't want to get into this important question of priority now – as someone who doesn't put the cart before the horse, but before the horse. People had always said before that the distribution of forces in space had to be studied in a certain way. Helmholtz turned the matter around. He said that one must study the universe in such a way that only the whole universe can be a perpetuum mobile, whereas no individual process in the universe can ever be a perpetuum mobile. The people before Helmholtz had tried to explain the world without any perpetuum mobile at all. But now Planck says that a similar process must occur with regard to the ether. There are countless theories about the ether, ranging from the idea that was held in the past, when the ether was thought of as rarefied matter, to the idea of Lord Kelvin or J. J. Thompson, who imagined the ether to be a rigid liquid - of course, it is not to be thought of as a liquid like water - all intermediate stages are represented. And now Planck says as a physicist: Physics will only be cured when we start from the principle that no conception of the ether gives a tenable physics that attributes material properties to the ether. That is the sentence that one of the most important physicists of the present day has uttered. This means that if the ether is to be a tenable basis for physics, only spiritual properties may be attributed to it. And from this it follows that today's physicists are urged to think of matter as holes surrounded by ether, which, however, must be imagined as having no material but only spiritual properties. So: holes surrounded by spiritual ether, that is what must be taken as a basis in order to arrive at a tenable physics. This is being prepared today; it exists.
Now one can raise the question: Yes, but where does that leave the possibility of establishing a materialistic world view when physicists talk about matter consisting of holes and the ether can only have spiritual properties? One is almost forced to say: there is no longer any matter, there are only holes in the spiritual ether, and matter cannot change its location without exerting an influence on the surrounding ether, a reaction in the spiritual ether. That is what physics comes to.
However, one will need a sharp logic and must not be afraid to tackle such questions as to how the concept of speed is really to be grasped if it is not to contradict what the experiment expresses.
Take these things as something that should be said to prove that the humanities, so reviled as being unscientific, are in their foundations infinitely more scientific than that which is considered science today, because they approach things, I would say get to grips with them, in the sharpest logic. And that is what we must seek above all: a sharp grasp of the concepts, a definite conception of what otherwise confronts us as a vague thing in the world.
Episodische Betrachtung Über Raum, Zeit, Bewegung
Ich dachte, es würden heute höchstens ein Dutzend da sein und wollte, wie es ja auch geschehen soll, etwas sagen, das ganz episodisch etwas gar nicht zu unseren sonstigen Betrachtungen Gehöriges sein soll, das aber für einige, die sich in die Sache etwas hineinleben können, wichtig sein kann zur Beurteilung von manchem, was in bezug auf gewisse Auffassungen von Raum und Zeit und Bewegung zur Zeit eine Rolle spielt.
Es gibt heute nämlich theoretische Physiker, die der Meinung sind, daß sich mit Bezug auf die einfachsten Weltvorstellungen eine tiefgehende Umwälzung vollziehe. Unter diesen einfachen Weltvorstellungen, die der theoretischen Physik zugrunde liegen, wollen wir eben heute ein klein wenig etwas betrachten, was sich auf Zeit, Raum und Bewegung bezieht. Es wird dies die Grundlage abgeben zu einer in der nächsten Zeit anzustellenden weitergehenden Betrachtung, die uns tiefer hineinführen kann in das, was man gerade in der Gegenwart bei grundlegenden physikalischen Betrachtungen anstreben will.
Sie werden ja gewiß alle schon davon gehört haben, daß sich in der Gegenwart dasjenige geltend macht, was man die Relativitätstheorie der neueren Physik nennt. Die Relativitätstheorie - es gibt da auch mancherlei Schattierungen - wird heute von zahllosen theoretischen Physikern vertreten. Man verspricht sich von ihr einen völligen Umschwung aller Begriffe, die von den Physikern, wenn sie eben elementare theoretische Betrachtungen angestellt haben, bisher als richtig anerkannt wurden und die ja im wesentlichen zurückgehen auf Newton. Nun glauben die neueren theoretischen Physiker von heute, daß alle diese Newtonschen Begriffe, die noch zu unserer Studentenzeit als ganz unumstößlich aufgenommen worden sind, eine Umwälzung erfahren müssen, ja, daß gewissermaßen die ganze theoretische Grundlage der Physik, wie sie geglaubt worden ist und noch geglaubt wird, eigentlich falsch sei. Nun, warum ich die Betrachtung, die ich anstellen will, in Zusammenhang bringen muß mit dieser neu auftauchenden Relativitätstheorie, das wird sich später ergeben.
Damit nun das, was ich zu sagen habe, nicht ganz unverständlich bleibe, möchte ich von ganz einfachen, elementaren Begriffen ausgehen, um Ihnen dutch dieselben gleich vorzuführen, was für eine Vorstellung man mit dem Zeitbegriff verbinden kann. Gehen wir, wie gesagt, von ganz elementaren Dingen aus. Nehmen wir einmal an, irgendein Objekt, das ich meinetwillen \(a\) nennen will, eine rollende Kugel oder dergleichen, bewege sich in einer Richtung, die ich durch diese Linie andeuten will; also \(a\) bewegt sich längs der Geraden in der Richtung nach \(b\):

Nun wissen Sie ja alle, daß man den Weg, die Weglänge, welche ein solches Bewegtes in einer Sekunde zurücklegt, die Geschwindigkeit nennt. Nehmen wir also an, \(a\) käme in einer Sekunde bis hierher, bis \(a_1\), dann würde man diese Wegstrecke \(a\) bis \(a_1\) in der Physik die Geschwindigkeit nennen und mit \(c\) bezeichnen. Und wenn wir des weiteren annehmen, daß das sich Bewegende durch die folgenden Sekunden weitergehe, so würde es, wenn es eine gleichförmige Bewegung vollführen würde - und nur von einer solchen wollen wir reden -, am Ende der zweiten Sekunde bei \(a_2\) sein, wobei \(aa_1 = a_1a_2\) ist, das heißt, mit derselben Geschwindigkeit \(c\) geht in der zweiten Sekunde das sich Bewegende von \(a_1\) nach \(a_2\) in der dritten Sekunde von \(a_2\) nach \(a_3\) in der vierten Sekunde von \(a_3\) nach \(a_4\) und so weiter fort. Nehmen wir nun an, wir betrachteten diese Bewegung eine gewisse Zeit hindurch und unser Bewegliches käme eine bestimmte Strecke weit, nehmen wit an bis \(a_5\)

dann nennt man, wenn dieses Bewegliche von \(a\) nach \(a_5\) gerollt ist, das Stück des Raumes - den wir hier in seiner einen Dimension auffassen — den Weg; so daß \(a\) bis \(a_5\) der Weg ist, den es zurückgelegt hat; \(c\) ist die Geschwindigkeit; den Weg bezeichnet man mit \(s\); und man sagt: das Bewegliche \(a\) habe den Weg \(s\) mit einer Geschwindigkeit \(c\) in einer bestimmten Zeit - hier fünf Sekunden - durchlaufen. Diese Durchlaufszeit bezeichnet man mit \(t\).
Nun gibt es eine bestimmte Beziehung zwischen Weg, Zeit und Geschwindigkeit. Die einfachste Beziehung, die man gefunden hat, ist die, daß man hier sagen würde: \(s\) - der Weg - ist fünfmal von \( \)bis \(a_1\), das heißt, einmal den Weg \(a\) bis \(a_1\) mal \(5\), das sind \(5\) Sekunden, das ist also die Zeit; also müssen wir das, was wir die Geschwindigkeit genannt haben - dieses Stück \(aa_1\) — mit \(5\) multiplizieren, dann bekommen wir den Weg \(s = c \cdot t\) (Weg = Geschwindigkeit \(\cdot\) Zeit). Es stecken also drei Begriffe in dieser Formel: \(s\), \(c\), \(t\).
Nun wissen Sie ja, daß über die Zeit von einer Anzahl von Philosophen, Mathematikern und auch theoretischen Mechanikern unendlich viel geschrieben worden ist. Die Menschen glauben zwar, von der Zeit eine Vorstellung, einen Begriff zu haben, aber es würde jeder, wenn er erklären müßte und nachdenken würde, was er unter Zeit versteht, sehr bald einsehen, daß er doch keine rechte Vorstellung von diesem Begriff der Zeit hat, der zu den allergangbarsten Begriffen gehört, die man in der Mechanik anwendet. Um nun irgend etwas über den Zeitbegriff studieren zu können, wollen wir uns an diese Formel halten, die ja zunächst den Zeitbegriff in eine gleichförmige, gradlinige Bewegung hineinversetzt. Aber wenn auch diese Formel in jedem Physikbuch steht, so ist sie in der Physik doch umspielt von einer ganzen Menge, ich will nicht sagen Unklarheiten, aber von mangelnder Klarheit, von wenig Willen, tiefer in die Sache hineinzugehen. Und das rührt namentlich davon her, daß in unseren Schulen der Unterricht in bezug auf etwas, das wir alle lernen, uns nicht gewisse Unterscheidungen beibringt, die aber wichtig sind, wenn man zu genaueren Begriffen in einer gewissen Richtung kommen will. Wir lernen ja in unseren Schulen von vier Rechnungsarten reden: von Addition, Subtraktion, Multiplikation und Division. Aber bei der Division werden wir, ich glaube, nicht oft darauf aufmerksam gemacht, daß in der gewöhnlichen Rechnungsoperation eigentlich zwei total verschiedene Dinge stecken. Ich will Ihnen das in ganz einfacher Weise zeigen.
Nehmen wir an, wir hätten einen gewöhnlichen Apfel und teilen diesen. Wir können ihn in fünf, in zehn Teile teilen und so weiter, dann bekommen wir, wenn wir ihn geteilt haben, einen so- und sovielten Teil des Apfels. Wollen wir die Teile verteilen, so ist das, was wir verteilen, eben ein Stück des Apfels. Wir führen hier wirklich eine Division aus. Ich will es als Bruch schreiben, denn das ist dasselbe wie eine Division. Ich kann sagen: Ein Apfel wird, sagen wir in zehn Teile geteilt, dann bekommen wir als Resultat ein Zehntel Apfel. Sehen Sie sich jetzt einmal an, was ich auf die Tafel geschrieben habe:
$$\frac{1 Apfel (Dingliche)}{10 (Zahl)} = \frac{1}{10}Apfel (Dingliches)$$In dem Zähler oder Dividenden haben wir eine Qualität, irgend etwas Dingliches; im Divisor oder Nenner haben wir nichts Dingliches, sondern eine bloße Zahl; \(10\) ist hier eine bloße Zahl; und im Quotienten haben wir wiederum etwas Dingliches: ein Zehntel Apfel.
Diese Sache ändert sich nicht, wenn wir statt einem Apfel zwanzig Äpfel teilen. Nehmen wir an, wir teilen \(20\) Äpfel durch \(10\), so bekommen wir statt ein Zehntel Apfel \(2\) Äpfel:
$$\frac{20 Apfel}{10} = 2 Apfel$$Die \(20\) Äpfel sind wiederum ein Dingliches; unten ist bloß die Zahl und als Quotient bekommen wir wiederum ein Dingliches. Das ist eine Division.
Aber das Dividieren kann noch einen ganz anderen Sinn haben. Ich kann oben im Dividenden \(20\) Äpfel haben, aber unten als Nenner oder Divisor, sagen wir \(2\) Äpfel, dann habe ich oben und unten ein Dingliches. Was bekomme ich da als Resultat? Dann bekomme ich als Resultat kein Dingliches, sondern ich bekomme heraus, wie oft \(2\) Äpfel in \(20\) Äpfel enthalten sind, ich bekomme \(10\), das heißt, ich bekomme eine Zahl:
$$\frac{20 Apfel (Dingliches)}{2 Apfel (Dingliches)} = 10 (Zahl)$$Wiederum habe ich es mit einer Division zu tun, aber diese hat jetzt einen ganz anderen Sinn als die Division im ersten Fall. Im ersten Fall teile ich ein Dingliches und bekomme wieder ein Dingliches, im zweiten Fall teile ich gar nicht, sondern stelle mir die Aufgabe, zu erforschen, wie oft ein Dingliches in einem anderen Dinglichen enthalten ist und da bekomme ich eine Zahl heraus.
Wir können also sagen: Division ist nicht immer Dividieren, sondern es gibt zwei Arten von Divisionen, die sich streng voneinander unterscheiden. Man müßte also beim Unterrichten immer auseinandersetzen, daß man zwei Arten von Divisionen hat. Bei der ersten stellt sich mir die Aufgabe, zu erforschen, was herauskommt, wenn man ein Dingliches teilt; bei der zweiten stellt sich die Aufgabe, zu erforschen, wie oft ein Dingliches in einem gleichartigen Dinglichen enthalten ist — sie müssen gleichartig sein, denn man kann natürlich nicht fragen, wie oft \(2\) Äpfel in \(20\) Birnen enthalten sind - und dann bekommen wir eine Zahl heraus.
Dies muß man ins Auge fassen, wenn man die Formel \(s = c \cdot t\) studieren will.
Nun kann diese Formel auch anders geschrieben werden. Ich brauche nicht immer das \(s\) zu suchen, sondern ich kann auch das \(c\) oder \(t\) suchen, dann ändert sich die Formel. Suche ich das \(c\), dann bekomme ich es, indem ich das \(s\) dividiere durch \(t\). Indem ich den ganzen Raum durch \(t\) dividiere, bekomme ich den Raum, der in \(5\) Sekunden durchmessen worden ist, durch \(5\), also die Geschwindigkeit \(c\): $$c= \frac{s}{t}$$ Ebenso können Sie aber \(t\) bekommen: die Zeit. Nehmen wir an, daß Sie \(s\) dividieren durch \(c\). Wenn Sie fragen: Wie oft ist in dem ganzen Weg der Weg von einer Sekunde enthalten, so ist er fünfmal enthalten. Da bekommen Sie die Zeit:
$$t = \frac{s}{c}$$Sehen wir uns diese Formeln genauer an. Nehmen wir zunächst die zweite und vergleichen wir: \(s\), das ist der Weg hier, die Länge \(a\) bis \(a_5\), das haben wir in dem Zähler; hier im Nenner haben wir das \(c\). Was ist das \(c\)? Nun, das ist der Weg in einer Sekunde. Wege sind das: \(s\) ist ein Weg, \(c\) ist ein Weg. Welcher Form von Division gleicht denn das? Nun, das gleicht dieser Form \(20\) Äpfel : \(2\) Äpfel = \(10\). Hier (im Zähler) haben Sie Äpfel und hier (im Nenner) haben Sie Äpfel; hier (im Zähler von \(\frac{s}{c}\)) haben Sie Weg und hier (im Nenner) haben Sie Weg. Was muß denn da vorne stehen? Bloß eine Zahl. Das heißt, \(t\) kommt bei unseren physikalischen Betrachtungen als nichts anderes heraus denn als eine Zahl. Denn wenn ich \(s\) und \(c\) betrachte als Weg, also als ein Dingliches - beide sind ja Weg oder ein Stück von einem Weg -, dann kann aus der Natur der Teilung die Zeit \(t\) nur figurieren als eine Zahl. Geradeso wie die Zahl \(10\) (\(20\) Äpfel : \(2\) Äpfel = \(10\)) eine Zahl ist und nichts weniger oder mehr, so kann in dieser Division \(t\), die Zeit, auch nichts anderes als eine Zahl sein.
Sie können auch die Divisionsform nehmen \(1 Apfel: 10 = \frac{1}{10} Apfel\), dann gleicht diese der Formel \(c = \frac{s}{t}\). Wird dagegen Dingliches dividiert durch Dingliches, was muß herauskommen? Eine Zahl wie hier \(t = \frac{s}{c}\), wo wit es bei \(t\) mit einer bloßen Zahl zu tun haben. Das heißt, beide Formeln weisen darauf hin, daß - insofern wir bei der Physik stehenbleiben - wir für die Zeit nach der Natur der Teilung nichts anderes herausbekommen als eine Zahl. Und zwar handelt es sich hier (\(20\) Äpfel: \(2\) Äpfel = \(10\)) um eine Zahl, die sich auf Äpfel bezieht und zeigt, wie oft \(2\) Äpfel in \(20\) Äpfeln enthalten sind, und hier bei der Zeit \(\frac{s}{c} =t\) um eine Zahl, die zeigt, wie oft die Geschwindigkeit im Raume enthalten ist.
Nun wird wohl niemand von Ihnen in der Zahl als solcher ein Dingliches sehen. Wenn Sie irgendeinem Buben oder Mädchen nicht \(3\) Äpfel geben, sondern bloß \(3\) als Zahl, so werden sie nicht satt. Also in der Zahl kann man nicht ein Dingliches sehen, sondern eben eine bloße Abstraktion, etwas, was bloß gewissermaßen Beziehungen angibt in der äußeren Welt.
Aus dieser Betrachtung können wir ersehen, daß uns die Zeit durch die physikalische Betrachtung selber aus der Hand entschlüpft; sie schrumpft uns zu einer bloßen Zahl zusammen. Ebensowenig wie wir über die Zahl philosophieren können, können wir auch nicht über die Zeit philosophieren, das heißt, sie hat sich auf die Vorstellung einer Zahl reduziert. Darum können wir auch die Zeit in den Dingen nicht finden, wenn wir noch so lange überall suchen, weil sie bloß als Zahl figuriert. Womit hängt das zusammen? Nun, ich glaube, ein Bub oder ein Mädchen braucht nicht besonders alt zu sein, um eine aus gesundem Gefühl hervorgehende Antwort zu geben, wenn man frägt: Was interessiert dich, die Äpfel oder die Zahl? Gewiß könnte jemand sophistisch reden und sagen, mich interessiert die Zahl, denn mir sind \(8\) Äpfel lieber als \(6\); aber das ist doch nur, weil \(8\) Äpfel mehr sind als \(6\). Also die Zahl ist gar nicht das, um was es sich ihm dabei handelt, sondern die Äpfel sind es, das Dingliche ist es.
Daraus aber folgt, daß wir uns überhaupt an das Dingliche halten müssen und uns nicht an die Zahl halten dürfen, wenn wir von Zeit, Raum und Geschwindigkeit sprechen. Und wenn wir nun das Dingliche ins Auge fassen, so fällt die Zeit von vornherein weg, das heißt, sie ist Zahl und nicht Dingliches. Sie werden sich also sagen können: Wir haben \(s\), den Raum, das Stück des Raumes, das unser Bewegliches durchläuft. Wenn das nun weiter rollt, so kann es noch viel, viel Raum durchmessen. Der Raum ist ja draußen etwas Dingliches. Das ist aber nicht das, worauf es zunächst ankommt, denn man kann sich den Raum als immer weitergehend denken. Aber etwas anderes hat sehr viel mit dem zu tun, worauf es uns ankommt, das ist das \(c\). Denn wie das \(a\) den Raum durchläuft, das hängt ganz davon ab, ob es in einer Sekunde, sagen wir, \(20\) oder \(25\) oder \(50\)cm und so weiter durchläuft, und wiederum, wieviel es durchläuft, das hängt davon ab, wie schnell es läuft. Aber wie schnell es läuft, das hat es im Innern, das ist ihm im Innern eigentümlich. Und von dem, was dem Beweglichen im Innern eigentümlich ist, hängt überhaupt der ganze Vorgang ab. Also auf die Geschwindigkeit des Beweglichen kommt es an, die gehört dem Beweglichen als solchem an, ist eine innere Qualität des Beweglichen. Und wenn wir die Welt anschauen, insofern wir sie auf mechanische Vorgänge hin betrachten, dann müssen wir, wenn wir von der Realität sprechen, von der innerlichen Geschwindigkeit der Kötper oder Atome oder Moleküle sprechen. Und der ganze Vorgang zwingt uns, von der innerlichen Geschwindigkeit als von zu den Dingen zugehörig zu sprechen, so wie der Rose die rote Farbe zugehörig ist.
Also der Fundamentalbegriff ist die Geschwindigkeit; sie ist das, worauf es ankommt. Darauf folgt, daß wir uns nicht an die Formel halten dürfen, die hier \(c\) hat \(c = \frac{s}{t}\), und nicht glauben dürfen, daß wir mit Raum und Zeit irgend etwas besonders Reales haben, sondern was real ist in den Dingen, das ist die Geschwindigkeit, nicht die Zeit. Die Zeit ist wiederum erst abstrahiert von dem Begriff der Geschwindigkeit, weil die Dinge verschiedene Geschwindigkeit haben. Blicken wir auf die verschiedenen Geschwindigkeiten und wollen sie auf ein Gemeinsames reduzieren, so bekommen wir den Begriff der Zeit. Dieser ist eine Abstraktion, ebenso wie der Gattungsbegriff «Apfel» eine Abstraktion ist und real nur der besondere, der konkrete Apfel ist. Wenn wir also auf das mechanisch Reale der Dinge eingehen, so müssen wir auf die Geschwindigkeit eingehen und dürfen nicht glauben, daß wir den Zeitbegriff in den Vordergrund stellen können. Das ist der große Fehler, der überall in der Physik gemacht wird, daß man nicht beachtet, daß man von der Geschwindigkeit ausgehen muß, die innen in den Dingen ist, die zu ihnen so gehört, wie das Leben zu den lebendigen Körpern.
Also halten Sie fest, meine lieben Freunde: nicht die Zeit, sondern die Geschwindigkeit ist dasjenige, was der Mechanik zugrunde liegen muß. Sie könnten nun sagen, das sind ja Spintisierereien, diese Unterschiede zu machen. Es sind aber keine Spintisierereien, sondern diese Dinge sind zur Auffassung gewisser Verhältnisse des Wirklichen fundamental bedeutsam und ich will Sie gleich auf etwas hinweisen, das zeigt, wie fundamental bedeutsam sie sind.
Bei den verschiedenen Diskussionen über die Relativitätstheorie handelte es sich bei den Leuten gerade darum, mit dem Zeit- und dem Geschwindigkeitsbegriff zurechtzukommen. Nun will ich Ihnen an zwei Spekulationen zeigen, in welcher Art gewisse Menschen denken, wie sie ihr Denken formulieren, wenn sie über Zeit und Geschwindigkeit reden. Da muß ich Ihnen eine merkwürdige Persönlichkeit vorführen, Herrn Lumen, der bei der Relativitätstheorie eine gewisse Rolle spielt. Was ist das für ein merkwürdiger Herr? Ja, sehen Sie, das ist eine, ich möchte sagen, «Phantasie-Bekanntschaft», die Flammarion gemacht hat. Dieser Herr Lumen hat eine sehr merkwürdige Fähigkeit, die wir uns etwa in der folgenden Weise klar machen können.
Sie wissen ja alle aus Ihrem Physikunterricht, daß das Licht eine gewisse Geschwindigkeit hat; es durchmißt in der Sekunde 300 000 km. c, also alles das, was dem Licht nach unserer Auffassung innerlich mechanisch angehört, das ist eine Geschwindigkeit von 300 000 km in der Sekunde. Nehmen wir zum Beispiel an, hier sei die Erde und von den Gegenständen und Vorkommnissen, die auf der Erde geschehen, geht in den Weltraum der Lichtstrahl hinaus (wurde an der Tafel schematisch angedeutet) und man sagt ja, weil das Licht hinausgeht, sieht man die Dinge. Nehmen wir nun das Folgende an. Wir haben hier jetzt diese etwas abstruse mathematisch-physikalische Stunde, und, sagen wir von drei bis vier Uhr gab es eine Eurythmiestunde. Von alledem geht in den Weltraum das Licht hinaus und man kann von draußen beobachten, was da hier geschieht. Und da das Licht mit einer Geschwindigkeit von 300 000 km in der Sekunde hinausgeht, so ging auch das, was heute nachmittag zwischen drei bis vier Uhr hier geschehen ist, mit einer Geschwindigkeit von 300000km in der Sekunde in den Raum hinaus, so daß, wenn Sie sich einen Beobachter denken, der 300 000km weit weg ist, dieser dasjenige, was auf der Erde hier geschieht, erst nach einer Sekunde sieht.
Nun nimmt Flammarion von jenem Herrn Lumen an, daß er noch schneller als das Licht, nämlich mit einer Geschwindigkeit von 400 000km in der Sekunde in den Weltenraum hinaussaust. Was wird die Folge davon sein? Er wird fortwährend das Licht überholen, denn nachdem das Licht eine Sekunde gegangen ist, ist er schon um 100 000 km weiter weg und er muß, wenn er da so heraussaust und zurückschaut, zu den Kundgebungen des Lichtes kommen, wo er das sieht, was hier jetzt und zwischen drei und vier Uhr geschehen ist. Da er aber das Licht nicht nur einholt, sondern überholt, so muß daraus folgen, daß er nicht zuerst die Eurythmiestunde und dann unsere Stunde wahrnimmt, sondern alles umgekehrt, zuerst das Ende und dann das Frühere. Es ist ein sonderbares Schauspiel, das dieser Herr Lumen erlebt. Alles sieht er so, daß er zuerst das Ende und dann den Anfang sieht, denn er überholt ja das Licht.
Solche Vorstellungen haben, wie gesagt, eine gewisse Rolle gespielt gerade bei den Diskussionen über die Relativitätstheorie. Noch eine andere Vorstellung möchte ich Ihnen vorführen, die auch eine gewisse Rolle gespielt hat und die sich der Naturforscher Baer gebildet hat. Er hat sich gesagt: Man könnte sich vorstellen, daß der Mensch sein Leben nicht in etwa 70 oder 80 Jahren, sondern in 70 oder 80 Sekunden durchlebt. Sein Puls müßte einfach um soviel schneller schlagen, daß in einer Sekunde ein Jahr enthalten wäre. Dadurch könnte bewirkt werden, daß der Mensch nicht einmal wie eine Eintagsfliege, sondern wie ein 70-Sekunden-Tier wäre, wenn nur sein Puls entsprechend schnell schlüge. Was würde die Folge sein? Solch ein Mensch würde in 70 Sekunden Ungeheures durchleben. Wenn er zum Beispiel eine Pflanze anschaut, die ihrer Art treu geblieben ist, so würde er niemals zu der Anschauung kommen, daß eine Pflanze aus der Erde herauswächst, sondern er würde zu der Anschauung kommen, daß Pflanzen ewige Gebilde sind. Also ganz anders würde ein solcher Mensch zur Welt stehen, einfach dadurch, daß die Geschwindigkeit seines Lebens in demselben Maße vergrößert zu denken wäre wie die Geschwindigkeit seines Pulsschlages im Vergleich zu uns anderen Menschen. Oder, sagt Baer, stellen wir uns vor, der Mensch lebe nicht 80 Sekunden oder 80 Jahre, sondern 80000 Jahre und der Pulsschlag ginge um so viel langsamer, dann würde die ganze Welt wiederum anders sein. Zum Beispiel würde dann die Sonne, während sie für uns mit einer gewissen Geschwindigkeit geht, über den Himmel rasen wie ein feuriger Wind; nicht die einzelne Sonne würde man unterscheiden, sondern sie würde herumrasen wie ein rötliches Rad. Pflanzen würden flugs aufschießen und mit rasender Geschwindigkeit wiederum vergehen und so weiter.
Das hat Baer hingestellt als einen möglichen Gedanken, um zu zeigen, wie das Weltbild von der subjektiven Konstitution des Organismus abhängt. Sie sehen, da kommt alles, alles ins Wanken.
Wenn man die Art des Denkens, die einer solchen Vorstellung wie der Flammarions von Herrn Lumen oder der von Baer zugrunde liegt, ins Auge faßt, so ist eines wichtig zu beachten. Nehmen wir noch einmal den Herrn Lumen. Es wird vorausgesetzt, daß Herr Lumen in der Lage wäre, 400 000 km in der Sekunde zu fliegen, also das Licht zu überholen und die späteren Lichtbilder einzuholen. Aber nun nehmen Sie einmal dasjenige, was Sie als wirklich nehmen können, wenn Sie auf unsere geisteswissenschaftlichen Begriffe tiefer eingehen. Wir können sogar ganz absehen von dem gröberen physischen Leib und gleich auf den Ätherleib eingehen. Ja, wenn wir auf den Ätherleib eingehen, was ist er denn? Er ist Äther, Lichtäther, er ist selber webendes Licht. Halten Sie das fest, denn was folgt daraus? Es folgt daraus doch, daß, wenn wir uns im Raume bewegen, wir uns im höchsten Falle mit der dem Licht eigentümlichen Geschwindigkeit bewegen können. Wenn also jemand sagt, ein Mensch wie Herr Lumen bewege sich mit einer Geschwindigkeit von 400 000km in der Sekunde, dann müssen wit fragen - ich will sogar den physischen Leib auslassen und nur annehmen, daß sich ein Ätherleib herausbewegen könnte -, wie schnell könnte er sich nur bewegen? Nun, höchstens mit einer Geschwindigkeit von 300000km in der Sekunde, mit der Lichtgeschwindigkeit. Vom Ätherleib kann man nicht sagen, daß er das Licht überholt, denn er ist selber bewegliches Licht. Also der Herr Lumen darf nicht aus irgend etwas gewoben sein, was es im Raum gibt; mit anderen Worten: Er ist eine unwirkliche Vorstellung, er ist ein reines Phantasiegebilde. Denn dem Dinglichen oder Wesenhaften in der Welt ist seine Geschwindigkeit immanent oder inhärent. Sie ist in ihm drinnen. Sie ist seine Eigenschaft. Wir können sie nicht herausreißen. Wir können gar nicht sagen: Wir sondern von dem Ding seine Geschwindigkeit ab -, sondern diese ist eine Eigenschaft des Dinges. Wir können nicht von einer Eigenschaft sprechen, die abgesondert außerhalb des Dinglichen liegt. So müssen wir auch gegenüber den Vorstellungen des Baer sagen: In dem Augenblick, wo man begreift, daß die Geschwindigkeit des Pulsschlages zum Dinglichen jedes Menschen gehört, begreift man auch, daß wir keine andere Geschwindigkeit als die unseres Pulsschlages haben können. Wir sind dadurch Mensch, daß wir eine gewisse Geschwindigkeit des Pulsschlages haben und wir können sie uns nicht beliebig denken, denn wir würden aufhören Mensch zu sein, wenn der Pulsschlag zum Beispiel tausendmal so schnell wäre, als er in Wirklichkeit ist. Die Geschwindigkeit gehört zum Dinglichen.
Es ist wichtig, zu sehen, wie Geisteswissenschaft zum Wesenhaften der Dinge führt, und wozu dasjenige Denken führt, das sich bis in unsere Zeit hinein entwickelt hat, ohne sich auf Geisteswissenschaft einzulassen. Es führt dazu, daß man sich Vorstellungen bildet wie die des Herrn Lumen oder die von dem tausendmal beschleunigten Pulsschlag, die schlechterdings unmöglich oder unreal sind. Man rechnet mit phantastischen Begriffen, wenn man nicht einsieht, daß die Zeit eine bloße Zahl ist. So hat die sogenannte rationelle Mechanik zu ganz irrealen Begriffen geführt. Geisteswissenschaft führt uns dazu zu sagen: Ja, was ist denn ein solcher Herr Lumen, der 400 000 km rast, während er höchstens 300000 ... [Lücke in der Nachschrift] ... Nichts anderes ist er als der berühmte Herr, der sich an seinem eigenen Schopf in die Höhe zieht.
Geisteswissenschaft ist also von diesem Gesichtspunkte aus dazu da, um das Denken des Menschen, das in die Phantastik geraten ist, wiederum in die Wirklichkeit zurückzubringen, es nicht von der Wirklichkeit abzubringen. Sie sehen, während man der Geisteswissenschaft vorwirft, daß sie phantastisch ist, ist sie in Wahrheit dazu da, um die phantastischen Vorstellungen und Begriffe der Physik zur Wirklichkeit zurückzuführen. Und es wird für ein gesundes Denken außerordentlich wichtig sein, daß in der Zukunft dem Gemüte der Kinder so etwas, wie die zwei Arten der Division wirklich beigebracht wird, so daß sie nicht mit allerlei Unklarheiten, sondern mit bestimmten Begriffen rechnen. Zu Vorstellungen und Begriffen, die eine Bedeutung für die Wirklichkeit haben, kann man nicht anders kommen, als daß man der Wirklichkeit eben wirklich gegenübertritt, das heißt, daß man mit Geisteswissenschaft denkt, denn da gehen einem reale, nicht phantastische Begriffe auf.
Die Physik hatte vor der Relativitätstheorie die Vorstellung Newtons, daß der Raum eine Leere ist, gleichsam ein Gefäß - unendlich oder nicht, das wollen wir jetzt nicht untersuchen - und die Zeit so dahinfließt wie ein gleichförmiger Strom; die Dinge sind im Raume drinnen und die Vorgänge verlaufen in der Zeit, und je nachdem ein Ding diese oder jene Zeit braucht, um einen bestimmten Raum zu durchmessen, erkennt man ihm eine gewisse Geschwindigkeit zu. Diese Vorstellung ist unwahr, weil sie gar nicht auf das Wesenhafte von Raum und Zeit sieht und dadurch die Geschwindigkeit, die eigentlich eine innere Eigenschaft ist, auseinanderlegt in die zwei irrealen Vorstellungen: Raum und Zeit. Die Geschwindigkeit ist wirklich das Ursprüngliche, während die Physik die Geschwindigkeit immer als eine Funktion von Raum und Zeit ansieht. Das was zu den Dingen gehört, ist aber das Wesenhafte, und Geisteswissenschaft zeigt, daß man gewisse Wege einschlagen muß, um nicht zu Phantasien über Raum und Zeit - wie der vom unendlichen Raum oder der von der Zeit als einem fortfließenden Strom - zu kommen, sondern zum wirklich Realen der Geschwindigkeit zu gelangen. Die ganze Mechanik, die wir in der Jugend aufgenommen haben als ein ungeheuer Sicheres, als das Sicherste, was es in der Wissenschaft gibt nach der Mathematik, sie operiert mit ganz vagen Begriffen, weil sie nicht weiß, welches die Natur der Geschwindigkeit ist und nicht weiß, diese als Fundamentales anzusehen.
Nun ist der Anstoß zur Relativitätsctheorie von Minkowski, Einstein, Planck, Poincaré, dem verstorbenen Mathematiker und Physiker und so weiter, gerade daher gekommen, weil sie nicht mehr zurechtkamen mit dieser kindlichen Newtonschen Vorstellung von dem leeren Raum und der regelmäßig fließenden Zeit und den Dingen, die sich mit einer gewissen Geschwindigkeit bewegen. Es ergaben sich aus gewissen Experimenten Begriffe, die nicht zusammenstimmten mit dem, was man als das Allersicherste angesehen hatte.
Nun habe ich Ihnen in der letzten Zeit hier einen Begriff entwickelt rein in Zusammenhang mit der Geisteswissenschaft, der vielleicht manchem überraschend gekommen sein mag. Ich habe den Begriff entwickelt, daß es gar nicht wahr ist, wenn man glaubt, in dem Kopf sei das Wichtigste die Substanz, die Materie, weil gerade da, wo wir Materie vermuten, es hohl ist und wir vom geisteswissenschaftlichen Standpunkte aus alle Hohlköpfe sind. Ich habe den Vergleich gebraucht mit den Luftperlen in einer Flasche Selterswasser. Da ist es auch so, daß da, wo wir glauben, etwas Reales, Wirkliches wahrzunehmen, nichts ist. Ringsherum ist das geistig Wirkliche und darin sind überall Löcher; die sieht man, so wie man auch beim Selterswasser nur die Blasen sieht, die Luft sind, das Wasser sieht man nicht. Und wenn die Leute glauben, daß da, wo ich anstoße an den Tisch, etwas sei, so stimmt das auch nicht, denn da ist eigentlich nichts. Ich stoße an den Hohlraum an und weil da nichts ist, darum kann ich eben nicht weiter.
Darauf sind wir aus geisteswissenschaftlichen Voraussetzungen ganz systematisch gekommen. Auf anderem Weg sind nun gewisse einsichtige und verständige Physiker zu ähnlicher Ansicht gedrängt worden, weil gewisse Vorgänge in der Natur sich einfach nicht vertragen mit den als so sicher geltenden Begriffen der Newtonschen Mechanik. Und zu diesen Dingen gehören zum Beispiel die Vorgänge an den Ihnen ja wohl bekannten Kathodenstrahlen, die man, wie Sie wissen, in gewissen evakuierten Glastöhren beobachten kann. Da hat man es zu tun mit etwas, das als Bewegliches Geschwindigkeit hat, mit Elektronen, bildlich gesprochen, mit fließender Elektrizität. Und durch die Beobachtung, durch das Experiment, zu dem die Physiker gekommen sind, indem sie in den Röhren die Kathodenstrahlen beobachtet haben, die fließende Elektrizität sind, kamen sie zu sehr eigentümlichen Vorstellungen. Und eine solche Vorstellung möchte ich Ihnen vorlesen. Sie findet sich in einem Vortrage von Poincaré über «Die neue Mechanik». Er knüpft da an die Vorstellungen an, die sich aus dem Kathodenstrahlen-Experiment ergeben, weil dieses namentlich nicht zusammenstimmt mit dem Newtonschen Begriff von Geschwindigkeit. Und da sieht er sich nach ziemlich wirren Gedankengängen zu folgendem Zugeständnis genötigt:... [Lücke in der Nachschrift] ..., und da fühlt sich der Physiker bewogen, folgendes zu sagen:
«Die Materie ist jetzt ganz passiv geworden. Die Eigenschaft, den Kräften, die ihre Bewegung zu ändern suchen, Widerstand zu leisten, kommt ihr im eigentlichen Sinne des Wortes nicht mehr zu. Wenn eine Kanonenkugel sich mit einer großen Geschwindigkeit bewegt und dadurch der Träger einer lebendigen Kraft, einer gewaltigen Energie wird, die Tod und Verderben ausstreut, so sind es nicht mehr die Eisenmoleküle, die den Sitz dieser Energie bilden, sondern dieser Sitz ist in dem Äther zu suchen, der die Moleküle umgibt. Man kann beinahe sagen, es gibt keine Materie mehr, es gibt nur noch Löcher im Äther.» - Nun, was wollen Sie mehr, meine lieben Freunde? -— «Und soweit diese Löcher eine aktive Rolle zu spielen scheinen, besteht sie darin, daß diese Löcher ihren Ort nicht verändern können, ohne den umgebenden Äther zu beeinflussen, der gegen dergleichen Veränderungen eine Reaktion ausübt.»
Materie sind Löcher im Äther! Die Physik ist also nach ihren heutigen Erfahrungen genötigt, dies einzugestehen. Und an solche Erfahrungen anknüpfend, hat ein anderer Physiker, Planck, einen Satz ausgesprochen, der höchst merkwürdig ist, nämlich den Satz, der besagt: Wir haben es erlebt in den vierziger Jahren des 19. Jahrhunderts, daß Helmholtz ein gewisses Problem so angefaßt hat - es war nicht Helmholtz, sondern Julius Robert Mayer, aber auf diese wichtige Prioritätsfrage wollen wir uns jetzt nicht einlassen —, wie der es tut, der nun das Pferd nicht am Schwanze, sondern am Kopf aufzäumt. Es hatten immer die Leute vorher gesagt, man muß die Verteilung der Kräfte im Raum in einer gewissen Weise studieren. Helmholtz hat die Sache umgedreht, er hat gesagt, man muß das Weltall so studieren, daß immer nur das ganze Weltall ein perpetuum mobile sein kann, während der einzelne Prozeß im Weltall niemals ein perpetuum mobile sein kann. Die Leute vorher hatten nämlich versucht, das Weltbild ganz ohne perpetuum mobile zu erklären. Nun aber sagt Planck, ein ebensolcher Vorgang müsse kommen bezüglich des Äthers. Über den Äther gibt es unzählige Theorien, angefangen von der Vorstellung, die man früher hatte, als man sich den Äther als verdünnte Materie vorstellte, bis zu der Vorstellung von Lord Kelvin oder J. J. Thompson, die sich den Äther als eine starre Flüssigkeit vorstellten - es ist natürlich nicht an eine Flüssigkeit wie Wasser zu denken -, sind alle Zwischenstufen vertreten. Und nun sagt Planck als Physiker: Die Physik wird erst dann gesund werden, wenn man ausgeht von dem Obersatze: Keine Vorstellung des Äthers gibt eine haltbare Physik, welche dem Äther materielle Eigenschaften beilegt. - Das ist der Satz, den einer der bedeutendsten Physiker der Gegenwart ausgesprochen hat. Das heißt also, daß dem Äther, wenn er eine haltbare Grundlage der Physik sein soll, nur spirituelle Eigenschaften beigelegt werden dürfen. Und daraus folgt also, daß die heutigen Physiker gedrängt werden, die Materie als Löcher zu denken und ringsherum den Äther, der aber so vorgestellt werden muß, daß er keine materiellen, sondern nur spirituelle Eigenschaften hat. Also: Löcher, von spirituellen Äther umgeben, das ist es, was zugrunde gelegt werden muß, um zu einer haltbaren Physik zu kommen. Das bereitet sich heute vor; das gibt es.
Nun kann man die Frage aufwerfen: Ja, wo bleibt dann noch die Möglichkeit, eine materialistische Weltauffassung zu begründen, wenn der Physiker davon redet, daß die Materie aus Löchern besteht und der Äther nur spirituelle Eigenschaften haben könne? Man muß also beinahe sagen: Es gibt keine Materie mehr, es gibt nur Löcher im spirituellen Äther und die Materie kann ihren Ort nicht verändern, ohne einen Einfluß auf den umgebenden Äther auszuüben, eine Reaktion im spirituellen Äther. Das ist es, wozu die Physik kommt.
Allerdings wird man eine scharfe Logik brauchen, wird sich nicht scheuen dürfen, solche Fragen anzugehen, wie der Begriff der Geschwindigkeit wirklich zu fassen ist, wenn er nicht dem widersprechen soll, was das Experiment ausdrückt.
Nehmen Sie diese Dinge als etwas, was gesagt werden sollte zum Beweis dafür, daß die als so unwissenschaftlich geschmähte Geisteswissenschaft in ihren Fundamenten unendlich viel wissenschaftlicher ist als dasjenige, was heute als Wissenschaft gilt, denn sie geht in schärfster Logik den Dingen, ich möchte sagen, zu Leibe. Und das ist es, was wir vor allen Dingen suchen müssen: ein scharfes Fassen der Begriffe, ein bestimmtes Auffassen dessen, was sonst als Vages in der Welt uns gegenübertritt.

